Mathematical Problem-Solving Style and Performance Of Students
- Luvie Jhun S. Gahi
- Ronald E. Almagro
- Richie Ryan C. Sudoy
- 1804-1820
- Dec 21, 2023
- Education
Mathematical Problem-Solving Style and Performance of Students
1Luvie Jhun S. Gahi, 2Ronald E. Almagro, 3Richie Ryan C. Sudoy
1Student, Master of Arts in Education Major in Mathematics, St. Mary’s College of Tagum,
2Student, Master of Arts in Education Major in English, St. Mary’s College of Tagum,
3Student, Doctor of Philosophy in Educational Management, Davao del Norte State College, Philippines
DOI: https://dx.doi.org/10.47772/IJRISS.2023.7011142
Received: 10 October 2023; Revised: 17 November 2023; Accepted: 20 November 2023; Published: 21 December 2023
ABSTRACT
This study aimed to determine whether the mathematical problem-solving style significantly affects the students’ performance in which a descriptive-correlational research design was used. Through stratified sampling, there were 291 first-year college respondents in the local college in Sto. Tomas, Davao del Norte who were chosen. This study used one adapted questionnaire and one researchers-made questionnaire with Mean, Pearson r, Standard Deviation, T-test, and Analysis of Variance used as the statistical tools. The students’ mathematics attained very good performance with the mathematical problem-solving style of students’ sensing, intuition, feeling, and thinking moderately observed. The findings revealed that the mathematical problem-solving style has a significant relationship with Students’ performance. However, there is a significant difference in the mathematical problem-solving style of students when grouped according to various programs. The result also revealed that there is no significant difference in the mathematical problem-solving style of students when grouped according to sex (male and female). Students, instructors, college administrators, and Commission of Higher Education officials (CHED) are encouraged to value the importance of mathematical problem-solving style in the performance of the students. Instructors, college administrators, and CHED officials must establish programs that will enhance the mathematical problem-solving styles and performance of students. College instructors and administrators must work collaboratively to achieve better performance in the mathematics. Therefore, these should ensure that the necessary materials, resources, activities, and differentiated instruction are provided and used to meet the students’ needs to learn and to encourage in the problem-solving style.
Keywords: Student’s Profile, Mathematical Problem-Solving Style, and Performance of Students
INTRODUCTION
Good problem-solving abilities are required for all issues emerging from daily activity or to progress through the developmental stages. Effective problem-solving style has been linked to beneficial psychological outcomes such as competence, productivity, and optimism (Carver & Scheier, 1999; Chang & D’Zurilla, 1996; Elliott, et al., 1994). Additionally, according to the National Council of Teachers of Mathematics (NCTM), problem-solving ability is an essential component of all mathematics learning. The ability to solve problems can provide significant benefits in everyday life and the workplace. However, problem-solving is not only a goal of learning mathematics, but also a major method of learning mathematical concepts.
Likewise, the process of problem-solving begins with the observation of a gap, the application, and the complete evaluation of a theory to close that loophole. Styles of problem-solving are viewed as contrasting individuals’ unique characteristics with the behaviors that people prefer to draw and concentrate on their efforts to arrive at some comprehension or awareness, generate ideas, and make plans for the work(Sutherland, 2002).In the local college of Sto. Tomas, Davao del Norte, the varying levels of student performance in mathematics courses was used in this study. Surprisingly, there seemed to be a gap in the published research addressing this issue (Almagro etal., 2023)
The ability and style to solve problems increase the students’ comfort level when solving mathematical problems and practical difficulties. In turn, having the ability to solve problems has several advantages. For instance, problem-solving style is a feature of mathematical activity and a key method for developing mathematical understanding (NCTM, 2000). This statement implies that problem-solving style is an essential component of mathematics education. Furthermore, students learn to apply their mathematical skills in various ways; they gain a deeper understanding of mathematical concepts and gain firsthand experience as a mathematician by solving problems (Badger et al., 2012). Consequently, instruction should be advanced to enable the students to recognize and address the issues they encountered in real-life situations (Phonapichat et al., 2014). Nevertheless, several research findings suggest that children struggle to solve problems because of (Herawatty et al., 2018). Thus, learning mathematics should encourage students to solve problems confidently using mathematics. Learning mathematics in school should assist the students in understanding and applying mathematics to their problems that occur in their daily lives and in the workplace. The learning program must enable students to develop new mathematical knowledge through problem-solving style, solve mathematics and other problems, implement and adjust various problem-solving strategies, and monitor and reflect on the problem-solving style (NCTM, 2000).
The existing literature acknowledges challenges in mathematical problem-solving, but there is a significant gap in understanding the specific difficulties students face and the effectiveness of different problem-solving strategies. GanzonandEdig (2022), recognize the challenges, there is a need for in-depth investigations into the categories of difficulties encountered during the problem-solving process, low academic performance during in the pandemic. Additionally, the literature notes the importance of problem-solving models (Foshay & Kirkley, 2003; Almagro & Edig,2023), but a gap exists in understanding the comparative effectiveness of these motivated learning strategies. Moreover, within Realistic Mathematics Education (RME), recognized for its real-world emphasis, there is a need to examine the specific contextual factors that contribute to or hinder students’ success in mathematical problem-solving. Addressing these gaps will provide valuable insights for supporting students in developing effective problem-solving skills in mathematics.
The objective of this study is to investigate the relationship between mathematical problem-solving styles and the performance of students. Specifically, it aims to identify the various problem-solving styles employed by students, explore the challenges they face in mathematical problem-solving, and assess the impact of different problem-solving strategies on overall performance. The study seeks to contribute valuable insights that can inform educational practices and enhance students’ proficiency in mathematical problem-solving.
Statement of the Problem
The purpose of this study was to determine the relationship between mathematical problem-solving style and performance of first-year college students in Sto. Tomas College of Agriculture, Science and Technology (STCAST)in the academic year 2022-2023.
Specifically, these research questions sought to answer the following:
1. What is the measurable level of students’ performance in solving math problems?
2. What is the quantifiable level of students’ mathematical problem-solving style, considering the dimensions of sensing, intuition, feeling, and thinking?
3. Is there a significant and measurable relationship between students’ mathematical problem-solving style and their performance?
4. Can the mathematical problem-solving style of students be significantly differentiated when grouped according to sex and program, making it a specific and measurable analysis?
5. What specific and measurable instructional interventions can be proposed based on the study’s results, ensuring relevance and time-bound applicability?
Hypotheses
The following hypotheses was tested at a 0.05 level of significance. Specifically, this was drawn to determine whether mathematical problem-solving style of students differ in terms of their sex and program.
- There is no significant relationship between the mathematical problem-solving style and performance of students.
- There is no significant difference on the mathematical problem-solving style of the students when classified according to sex and programs.
Theoretical Framework
The study is grounded in the original problem-solving style model, rooted in the concept of psychological functions as proposed by Jung (1923) and further developed by Moon (2008) and Taylor & Mackenny (2008). This model encompasses thinking, feeling, sensation, and intuition as the four psychological functions (Ghodrati et al., 2014). Building upon this foundation, the research draws attention to gender-specific problem-solving tendencies, with Burkey and Miller (2005) finding that women often employ intuition in work settings, contrasting the emphasis on rational problem-solving linked to masculinity by Wang, Heppner, and Berry (2007). Additionally, Conner (2000) identifies women as more intuitive global thinkers, emphasizing simultaneous, interconnected processing of information. The study aligns with the belief that students’ problem-solving methods significantly influence their academic achievement and success (Poshtiban, 2007; Morton, 2001).
Conceptual Framework
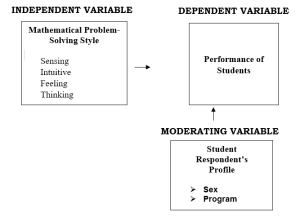
The study’s conceptual paradigm, which is shown in Figure 1, summarizes the variables which composed of mathematical problem-solving style and performance of students. On the one hand, the independent variable consists of mathematical problem-solving style which includes the indicators of sensing, intuitive, feeling, and thinking. On the other hand, the dependent variable consists of the performance of students in mathematics which composed of the moderating variable, i.e., the student respondent’s profile which are classified into sex and program. The researchers’ interpretation explains what the study wants to achieve as emphasized in the figure below. As such, the study aims to assess the Mathematical Problem-Solving Style of College students, and its relationship to their performance, and produce Students’ Instructional Intervention Plan that will improve their styles in solving mathematical problems.
Figure 1. The Conceptual Paradigm of the Study
METHODOLOGY
This section covers the study’s numerous methodologies, which include the research design, respondents, research instrument, data gathering procedures, statistical treatment of data, and ethical considerations.
Research Design
This study employed descriptive and correlational study design. Descriptive research entails gatherings of quantitative data that may be tabulated along a scale in numerical forms, such as test scores. It entails collecting data by describing occurrences and then arranging, tabulating, displaying, and summarizing the data (Glass & Hopkins, 1984). The researcher will utilize this design to determine and describe the variables employed in this study. It utilized the mean test since this aimed to measure the level of performance of students.
Correlational study, meanwhile, tried to establish correlations between two or more variables. It looked to see if a rise or drop in one variable corresponded to an increase or decrease in another (Tan, 2014). This design will be utilized by the researcher to examine and determine the existing correlations between the variables in this research.
This study was concerned with data collection utilizing adopted research instrument and a pilot-tested researchers’ made examination to evaluate the hypotheses whether the mathematical-problem solving style influences the student performance. It will test the data using the proper statistical tools. Furthermore, the study’s major objective is to distinguish between the mathematical problem-solving styles of students when they are classified by sex and program. Thus, the study intends to look into the relationship between mathematical problem-solving style and performance of freshmen students in various programs at Sto. Tomas College of Agriculture, Sciences, and Technology.
Participants of the Study
The respondents of this research were the first-year college students enrolled in bachelor of Technical and Vocational Teacher Education (BTVTED), Bachelor of Science in Agricultural Business (BSAB), Bachelor of Science in Office Administration (BSOA), and Bachelor of Public Administration (BPA)programs for the school year 2022-2023. The respondents’ total population size of this study comprises of 1,188 students coming from four (4) programs in Sto. Tomas College of Agriculture, Sciences and Technology (STCAST). Specifically, BSOA department consists of 426 first-year students, BSAB department consists of 381 first-year students, BPA department consists of 217 first-year students, and BTVTED department consists of 164 first-year students. By using Qualtrics online sample size calculator, given the identified collective population size of 1,188 students, the ideal sample size of this quantitative study will consist of 291 students in total.
Moreover, this study utilized a stratified sampling technique to determine the sample size and determine the final total number of respondents. As a result, the BSOA program has an ideal sample of 176 students, BSAB program with 94 students, BPA with 53 students, and BTVTED with 40 students.
Materials/Research Instrument
One adapted research instrument and one researcher-made examination were used in this study. This was selected and modified to match the overall objectives of the study. These research instruments were validated by a panel of experts.
Problem-Solving Style Questionnaire (PSSQ).This instrument contains a 20-item survey questionnaire comprising the six (4) components problem-solving in mathematics such as Sensing (5 items), Intuitive (5 items), Feeling (5 items), and Thinking (5 items). This questionnaire was anchored on a 5-point Likert scale ranging from 5 as strongly agree to 1 as strongly disagree.
The following parameter limits, with its corresponding descriptions, were applied for the level of students’ mathematical problem-solving style.
| Parameter Limits | Descriptive Equivalent | Interpretation |
| 4.20 – 5.00 | Very High | This indicates that mathematical problem-style is very much observed. |
| 3.40 – 4.19 | High | This indicates that mathematical problem-style is observed. |
| 2.60 – 3.39 | Moderate | This indicates that mathematical problem-style is moderately observed. |
| 1.80 – 2.59 | Low | This indicates that mathematical problem-style is less observed |
| 1.00 – 1.79 | Very Low | This indicates that mathematical problem-style is least observed |
The instrument for performance of students in Mathematics was a pilot-tested researcher-made questionnaire worth 40-item questionnaire. This instrument had been determined to possess good psychometric validity and reliability. The value of Cronbach’s a for the total scale is 0.747. All items of problem-solving skills are acceptable.
The percentage of the test score was computed by dividing the number of correct responses over the total highest possible score by multiplying it by 70 add 30. The highest probable score to achieve will be 40.
For the level of the mathematical problem-solving skills, the following parameter was used.
| Parameter Limits | Descriptive Equivalent | Interpretation |
| 80.01 – 100.00 | Exceptional | This indicates that the performance of students in mathematics is excellent. |
| 60.01 – 80.00 | Above Average | This indicates that the performance of students in mathematics is very good. |
| 40.01 – 60.00 | Average | This indicates that the performance of students in mathematics is good. |
| 20.01 – 40.00 | Poor | This indicates that the performance of students in mathematics is low. |
| 0.00 – 20.00 | Very Poor | This indicates that the performance of students in mathematics is verypoor. |
Data Gathering Procedure
The necessary data was gathered in a systematic procedure, which will involve the following.
Seeking permission to conduct the study. The researcher sought approval to conduct the research project. Primarily, the researcher will acquire a letter of recommendation from the College President of Santo Tomas College of Agriculture, Sciences, and Technology (STCAST). After acceptance, the researcher submitted a copy of the recommendation to the respective Department Heads of the following four (4) Degree Programs such as Bachelor of Science in Agriculture and Business (BSAB), Bachelor of Technical Vocational Teacher Education (BTVTED), Bachelor of Science in Office Administration (BSOA), and Bachelor of Public Administration (BPA) to finalize the approval to conduct the entire study.
General orientation and seeking of consent from research respondents. The study’s conduct was to regulate by ethical values, i.e., respect for individuals, beneficence, and justice, particularly in terms of data privacy and protection. Prior to data collection, the researcher will generate informed consent or assent forms and request them from respondents by e-mail. As evidence of their voluntary involvement in the full study, all forms will be delivered and signed electronically by those research respondents through e-mail message.
In addition, the researcher gave a brief 30-minute virtual presentation about the findings to the respondents. In accordance to this procedure, respondents who confirmed their voluntary involvement in the study were given a unique connection to Google Classroom developed by the researcher where the participants can partake in a brief virtual presentation. This was done specifically before conducting the survey. However, those respondents who were unable to attend the orientation due to unforeseen circumstances or personal reasons were educated about the research by phone call or chat through Facebook Messenger by the researcher. In addition, all respondents received a recorded video from the virtual presentation, which can be observed within the Google Classroom built by the researcher for the research.
Administration and retrieval of the questionnaire. The study took place in February of the school year 2022-2023. In order to carry out the research, the researcher will first develop Google Forms that will be utilized to collect responses from respondents based on the survey questions from the questionnaires. The quantitative data for this study was collected online using Google Forms. The researcher managed all direct contact and administration of surveys to respondents.
All surveys were allotted within one 90-minute session commencing with the Mathematical Problem-Style Questionnaire (MPSQ) and researcher-made examination. To protect the data, respondents was required to take the survey at a location and on a technological device (e.g., laptop, cellphone, or tablet) where only they have access to those offered online survey surveys via Google Forms. This was explicitly stated in their Informed Consent Form (ICF). In addition, the data questionnaire was returned to the researcher on time. Furthermore, the researcher handled personal communication and questionnaire administration. Finally, questionnaires were administered when the respondents’ 90-minute session has expired.
Checking, collating, and processing of data. The researcher gathered, validated, and quantified the respondents’ scores collected in an Excel file throughout this step. Following the tabulation, the data were submitted to an expert or certified statistician for data analysis. The researcher analyzed the results based on the data analysis for specific discoveries, discussions, and conclusions. This was accomplished mostly through data table and graphical presentations. Furthermore, descriptive statements were used to further explain and easily grasp the findings in relation to the study’s variables.
Statistical Tool for Data Analysis
The study’s findings were examined and comprehended properly using statistical methods such as Mean, Standard Deviation, Pearson r, T-test, and Analysis of Variance (ANOVA).
Mean. This method of analysis was used to measure the level of performance of students and their mathematical problem-solving style. Specifically, this was addressed in the first and second research questions.
Standard Deviation. A standard deviation is a statistical measure of the dispersion of a dataset in reference to its mean. This kind of analysis was used to determine how widely scattered the data is or how close the scores are to the mean. This was specifically answer the first and second research questions.
Pearson r. This statistical analysis was utilized to establish the existence of a significant relationship between mathematical problem-solving style and the performance of students. This will be utilized to specifically address the third research question.
T-test. This statistical analysis was utilized to determine if there was significant difference in the mathematical problem-solving style of students when classified into sex. This will specifically answer the fourth research question.
Analysis of Variance. This statistical analysis was utilized to determine if there was a significant difference in the mathematical problem-solving style of students when classified into four (4) different programs.
RESULTS AND DISCUSSION
In this chapter, the researchers present the results and discussions from the data gathered. In particular, this shows the data in tables and its corresponding descriptive interpretations.
Level of Mathematical Problem-Solving Style of Students in terms of Sensing
Table 1 presents the level of Mathematical Problem-Solving Style of Students in terms of Sensing. The item “As a student, I like to solve math problems and I am comfortable to trying to learn new skills.” has the highest mean of 3.69 with a descriptive equivalent of high. This is followed by the item “Before I put energy into solving math problems, I want to know first the benefits I can get from it.”, with a mean of 3.62 and high descriptive equivalent. On the contrary, the item “I tend to focus on immediate problems and let others worry about the distant future.” with the lowest mean of 3.20 and descriptive equivalent of moderate.
Table 1 Level of Mathematical Problem-Solving Style of Students in terms of Sensing
| Items | SD | Mean | Descriptive Equivalent |
| 1. In solving math problems, most people agree that I am an intellectual person. | 0.89 | 3.28 | Moderate |
| 2. I tend to focus on immediate problems and let others worry about the distant future | 0.98 | 3.20 | Moderate |
| 3. Before I put energy into solving math problems, I want to know first the benefits I can get from it. | 0.98 | 3.62 | High |
| 4. As a student, I like to solve math problems and I am comfortable to trying to learn new skills. | 1.02 | 3.69 | High |
| 5. As a student, I like theoretical and futuristic concepts. | 0.99 | 3.30 | Moderate |
| Category | 0.97 | 3.42 | High |
Furthermore, it has a category mean of 3.42 with descriptive equivalent of high. This indicates that the mathematical problem-solving style of students in terms of sensing is observed. Moreover, it has an Standard Deviation (SD) of 0.97.
The dispersion of the mathematical problem-solving style of students in terms of sensing based on the answers of the students revealed that the SD is 0.97. This indicates that the measures of variability of sensing as a mathematical problem-solving style of students are near the mean.
The result shows that students are interested in solving math problems and they are comfortable in trying new skills. It is also much observed that before solving math problems, students want to identify first the benefits they can get from it. Furthermore, students focus on immediate problems. Moreover, Vicente et al. (2002) observed that individuals with a sensation-type problem-solving style tend to focus on details and gather specific, factual data from their environment using their five senses. This approach involves a preference for concrete, practical, and tangible information, rather than abstract or theoretical concepts. These individuals tend to use a step-by-step approach to problem-solving, relying on established rules and procedures, and often prefer to work with real-world problems that have clear and immediate applications. Similarly, the study of Hsieh and Lin (2006) investigated the connection between mathematical problem-solving style and sensory preference among high school students. The study established that students with a sensing preference tended to use a more practical, sequential, and concrete problem-solving approach.
Level of Mathematical Problem-Solving Style of Students in terms of Intuitive
Table 2 presents the level of Mathematical Problem-Solving Style of Students in terms of Intuitive. The item “As a student, I solve math problems accurately by knowing all the details of the problem.” has the highest mean of 3.68 with a descriptive equivalent of high. This is followed by the item “As a student, I enjoy solving mathematical problems.” with a mean of 3.36 and a descriptive equivalent of moderate. On the contrary, the item “As a student, I solve mathematical problems quickly without wasting a lot of time on details.” has a mean of 3.05 with a descriptive equivalent of moderate.
Table 2 Level of Mathematical Problem-Solving Style of Students in terms of Intuitive
| Items | SD | Mean | Descriptive Equivalent |
| 1. As a student, I solve math problems accurately by knowing all the details of the problem. | 0.93 | 3.68 | High |
| 2.I’m am interested in long-range implications. | 0.95 | 3.13 | Moderate |
| 3. As a student, I solve mathematical problems quickly without wasting a lot of time on details. | 1.03 | 3.05 | Moderate |
| 4.As a student, I prefer to deal with new and complicated mathematical problems. | 1.06 | 3.09 | Moderate |
| 5.As a student, I enjoy solving mathematical problems | 1.06 | 3.36 | Moderate |
| Category | 1.01 | 3.26 | Moderate |
Furthermore, it has a category mean of 3.26 with a descriptive equivalent of moderate. It means that the mathematical problem-solving style of students in terms of intuitive is moderately observed. Moreover, the standard deviation of 1.01 in the category mean indicates that the measures of the variability of the mathematical problem-solving style of students in terms of intuition are near the mean.
It is observed that the students solve math problems accurately by knowing all the details of the problem. Additionally, it is moderately observed that students enjoy solving mathematical problems quickly and without wasting a lot of time on details. Hafriani (2018) suggests that students rely heavily on their intuition when it comes to solving mathematical problems. Students who use intuitive thinking to solve mathematical problems exhibit several characteristics: directness, self-evidence, intrinsic certainty, perseverance, coercion, extrapolation, globality, and implicitness.
Similarly, in the study conducted by Wuryanieet al., (2020) they found that students tend to rely on intuition when solving problems, exhibiting traits such as directness, self-evidence, extrapolation, intrinsic certainty, coercion, and decisiveness.
Level of Mathematical Problem-Solving Style of Students in terms of Feeling
Table 3 presents the level of Mathematical Problem-Solving Style of students in terms of feeling. The item “I want to solve math problems within a group and not individually.” has the highest mean of 3.73 with a descriptive equivalent of high. This is followed by the item “As a student, I can tell how others feel about solving math problems.” with a mean of 3.60 and a descriptive equivalent of high. On the other hand, the item “I try to please others and need occasional praise for myself.” has the lowest mean of 3.05 with a descriptive equivalent of moderate.
Table 3 Level of Mathematical Problem-Solving Style of Students in terms of Feeling
| Items | SD | Mean | Descriptive Equivalent |
| 1. As a student, I solve math problems emotionally and with motivation. | 0.90 | 3.46 | High |
| 2. I try to please others and need occasional praise for myself. | 1.05 | 3.05 | Moderate |
| 3. I want to solve math problems within a group and not individually. | 1.03 | 3.73 | High |
| 4. As a student, I can tell how others feel about solving math problems. | 0.96 | 3.60 | High |
| 5. As a student, I prefer harmony in a work group—otherwise efficiency suffers | 0.94 | 3.58 | High |
| Category | 0.98 | 3.48 | High |
Moreover, it has a category mean of 3.48 with a descriptive equivalent of high. It implies that the mathematical problem-solving style of students in terms of feeling is observed. Consequently, the standard deviation of 0.98 in the category mean indicates that the measures of variability of the mathematical problem-solving style of students in terms of feeling are close to the mean.
Based on the results, it is observed that students want to solve math problems within a group and not individually. In addition, it is also observed that students can tell how others feel about solving math problems. It is moderately observed that in solving math problems, students try to please others and need occasional praise for their selves. A study by Goez et al. (2005) found that students must gain information and abilities related to feelings. Moreover, Altun (2003) examined the students who tend to rely on their feelings when solving problems prioritize their emotional and personal approaches in the problem-solving process. Along with this, Ahmed et al. (2014) stated that the mathematical problem-solving style has a favorable effect on the student’s attention, motivation to learn, choice of learning tools, self-regulation of learning, and academic performance.
Level of Mathematical Problem-Solving Style of students in terms of Thinking
Table 4 presents the level of mathematical problem-solving style of students in terms of thinking. The item “As a student, I don’t let mathematics word problems discourage me, no matter how difficult they are.” has the highest mean of 3.65 with a descriptive equivalent of high. This is followed by the item “I solve math problems by analyzing all the facts and putting them in systematic order.” with a mean of 3.56 with a descriptive equivalent of high. On other hand, the item “When I have a math problem to be solved, I solve it, even if others’ feelings might get hurt in the process.” has the lowest mean of 3.08 with a descriptive equivalent of moderate.
Furthermore, it has a category mean of 3.36 with a descriptive equivalent of moderate. This implies that the mathematical problem-solving style of students in terms of intuitive is moderately observed. Consequently, the standard deviation of 1.01 in the category means indicates that the measures of variability of the mathematical problem-solving style of students in terms of intuition are close to the mean.
Table 4 Level of Mathematical Problem-Solving Style of Students in terms of Thinking
| Items | SD | Mean | Descriptive Equivalent |
| 1. As a student, I solve math problems objectively and logically. | 0.91 | 3.42 | High |
| 2. I solve math problems by analyzing all the facts and putting them in systematic order. | 1.00 | 3.56 | High |
| 3. When I have a math problem to be solved, I solve it, even if others’ feelings might get hurt in the process | 1.03 | 3.08 | Moderate |
| 4. As a student, I don’t let mathematics word problems discourage me, no matter how difficult they are. | 1.06 | 3.65 | High |
| 5. It is easy for me to make hard decisions. | 1.05 | 3.10 | Moderate |
| Category | 1.01 | 3.36 | Moderate |
Based on the results, it is observed that students approach mathematics word problems in a thoughtful and analytical manner. They are not easily discouraged by the difficulty of the problems and instead persevere until they find a solution. Students employ a systematic approach to problem-solving, carefully considering all the relevant facts and putting them in a logical order. This approach aligns with the findings of Khan et al. (2016), who emphasize the importance of analysis and research in effective problem-solving. Additionally, students demonstrate a commitment to objectivity and impartiality, seeking solutions that are grounded in evidence and reason. This aligns with the notion that mathematical problem-solving requires a high level of reflective thinking, as highlighted by Kneeland (2001) and Macaso and Dagohoy (2022). Moreover, the results of this study suggest that students are generally well-equipped to tackle mathematics word problems. They possess the necessary skills and mindset to approach these problems in a thoughtful, analytical, and objective manner. Thinking based on a mental process is an essential component of problem-solving solving, and problem-solving abilities are dependent on the correct application of thinking and solution processes. Furthermore, high-level thinking skills are involved in the intricate process of problem-solving (Gürsan & Yazgan, 2020).
Summary of the Level of Mathematical-Problem Solving Style of Students
Table summarizes the level of mathematical problem-solving style of students. Among the four indicators, “feeling” acquire the highest mean of 3.48 with descriptive equivalent of high. “Sensing” developed a mean of 3.42 with a descriptive equivalent of high. They have an SD of 0.98 and 0.97, respectively. It is followed by “thinking” with a mean of 3.36 with a descriptive equivalent of moderate and an SD of 1.01. On other hand, “intuitive” got the lowest mean of 3.26 with a descriptive equivalent of moderate and an SD of 1.01.
Table 5 Summary of the Level of Mathematical-Problem Solving Style of Students
| Indicators | SD | Mean | Descriptive Equivalent |
| 1. Sensing | 0.97 | 3.42 | High |
| 2. Intuitive | 1.01 | 3.34 | Moderate |
| 3. Feeling | 0.98 | 3.48 | High |
| 4. Thinking | 1.01 | 3.36 | Moderate |
| Overall Mean | 0.99 | 3.38 | Moderate |
Furthermore, it has an overall mean of 3.38 with a descriptive equivalent of moderate. This means that the mathematical problem-solving style of students is moderately observed. The findings suggest that there is a high degree of homogeneity in the mathematical problem-solving styles of the students, as evidenced by the small standard deviation of 0.99 in the overall mean. This indicates that the measures of variability in the students’ responses are clustered closely around the mean. Such a narrow range of variability in the problem-solving styles implies that the students have similar levels of proficiency in this variable.
Particularly, the results suggest that “feeling and sensing” as students’ mathematical problem-solving style is observed. This means that students want to solve math problems within a group and they like to solve math problems and are comfortable trying to learn new things. Moreover, “thinking and intuitive” as students’ mathematical problem-solving style is moderately observed. This indicates that students solve math problems by analyzing all the facts and knowing all the details of the problem.
This finding is supported by a recent study by TIMSS and PISA, which found that students can use their mathematical understanding and knowledge to solve problems (IEA, 2016). PISA assesses students’ ability to use their knowledge and skills in recognizing, analyzing, and solving problems in a variety of situations(OECD, 2019). Moreover, the result confirms the findings of Schoenfeld (2013), who mentioned that the mental state of students is an essential aspect of learning mathematics. The belief system of the student regarding himself, mathematics, and problem-solving determines student progress in problem-solving. This is also agreed by Hendriana et al., (2017) who mentioned that one of the fundamental mathematical abilities that students who study mathematics must develop is the ability to solve problems.
Level of Performance of Students in Solving Math Problems
Table 6 depicts the level of performance of students in solving math problems. The level of performance of students in terms of answering the 40-item Mathematics test has a mean of 60.84 with a descriptive equivalent of above average. This indicates that the performance of students in mathematics is very good. Furthermore, it has an SD of 10.48. This demonstrates whether one’s scores on mathematical problem-solving style were extremely high or extremely low. This suggests that students’ capacity to solve mathematical problems is more likely to deviate from the mean.
The result shows that the students were able to select and correctly identify the appropriate answer to the given questions. They could choose the correct equation from the problem and accurately determine the answer in the problem scenario. Additionally, they were able to verify their responses by selecting the correct answer to the given problem.
Table 6 Level of Performance of Students in Solving Math Problems
| Indicator | SD | Mean | Descriptive Equivalent |
| 1. 40-item Mathematics Test | 10.48 | 60.84 | Above Average |
As cited by Heris and Sumarmo (2014), problem-solving style are basic mathematical skills students need to learn. Fernandez et al. (2017) also added that problem-solving is a significant part of learning, making it of particular importance for the study of mathematics. Furthermore, another component of learning mathematics is problem-solving. This means that students need to become proficient in a variety of problem-solving style in mathematics in order to improve their creativity, reasoning, critique, and systematic thinking (NCTM, 2000). Therefore, completing mathematical problems is a crucial component of the learning objectives that must be encountered (Surya et al., 2017).
Significance of the Relationship Between the Variables
Table 7 presents the relationship between Mathematical Problem-Solving Style and the Performance of Students in Mathematics.
The correlation of Mathematical Problem-Solving Style has a significant relationship with the Performance of Students in Mathematics (p<0.05) with a coefficient determination of 0.733. Specifically, there is a strong positive correlation between the variables, and the p-value of the two variables is less than the 0.05 level of significance, which indicates that there is a significant relationship between the mathematical problem-solving style and the performance of students in mathematics (r=0.733,p=0.000. Thus, the null hypothesis is rejected.
Since the result confirmed that the mathematical problem-solving style and performance of students have a very high relationship, this means that the mathematical problem-solving style of the students significantly affects their performance in math. It can be seen from the aforementioned discussion that the mathematical problem-solving style of students is an important factor that affects their performance.
Table 7 Significance of the Relationship Between the Variables
| Variables Correlated | r | p-value | Decision on Ho | Decision on Relationship |
| Mathematical Problem-Solving Style and Performance of Students | 0.733 | 0.000 | Reject | Significant |
It can be deduced that if students’ mathematical problem-solving styles will be developed, then it enhances their performance in school. This is supported by the study conducted by Suratno et al., (2020) which found that there is a significant positive correlation between students’ problem-solving styles and academic performance of students. Additionally, Mustafić et al. (2017) established that a student would perform exceptionally well in any science subject if they propose a high level of self-concept toward problem-solving skills.
The significant difference in the Mathematical Problem-solving Style of students when grouped according to sex
To determine if there was a significant difference in the mathematical problem-solving style of students when grouped according to sex (male and female), a t-test was used. Table 8 shows the result.
Using the t-test, the obtained t-value is -2.211 and the resulting p-value is 0.9860. This result indicates that the difference in mathematical problem-solving style between males and females is not statistically significant at the 0.05 level. Therefore, the researchers fail to reject the null hypothesis that there is no significant difference in mathematical problem-solving style between male and female students.
Table 8 The significant difference in mathematical-problem solving style of students when grouped according to sex
| Moderating Variable | t-value | p-value | Decision on @ a=0.05 | Decision on Ho |
| Male | -2.211 | 0.9860 | Not Significant | Failed To Reject |
| Female |
This means that students’ mathematical problem-solving style when grouped according to sex (male and female) does not vary. This finding is consistent with previous research conducted by Rusdiet al., (2020) who mentioned that men and women have similar mathematical problem-solving styles. Male and female students may understand the information, describe their knowledge, and ask relevant questions. They can use notations, symbols, and mathematical models to describe the problem and solution properly. Moreover, Goos et al. (2017) studies examined the impact of gender differences on students’ mathematical learning outcomes have yielded inconsistent results. While some studies have demonstrated differences between genders, indicating that either men or women perform better, other studies have found no significant gender differences.
The significant difference in mathematical problem-solving style of students when grouped according to Program
Table 9 presents the significant difference in the mathematical problem-solving style of students when grouped according to four different programs: BTVTED, BSAB, BSOA, and BPA.
The result shows that there is a positive significant difference in the mathematical problem-solving style of students when grouped according to different programs since the f-value is 12.46 and the p-value is 0.000 which is lesser than 0.05 alpha level of significance. It indicates the mathematical problem-solving style of students from BTVTED, BSAB, BSOA, and BPA is significantly different. Therefore, the null hypothesis is rejected.
Table 9 The significant difference in mathematical problem-solving style of students when grouped according to Program.
| Program | Mean | F-value | P-value | Decision on Ho |
| BTVTED | 3.419 | 12.46 | 0.000 | Reject |
| BSAB | 3.644 | |||
| BSOA | 3.137 | |||
| BPA | 3.374 |
The result means that students coming from four programs have different problem-solving styles in mathematics. This finding is concurrent to the first model of problem-solving styles based on the concept of psychological functions (Jung, 1923; Moon, 2008; Taylor & Mackenny, 2008). This model consists of four psychological functions as thinking, feeling, sensation, and intuition (Ghodrati et al., 2014). As such, Hacısalihlioğlu et al. (2003)stated that achieving success in problem-solving is linked to possessing abilities such as critical thinking, decision-making, reflective thinking, inquiring, analyzing, and synthesizing. This is further confirmed by Wen-Chun et al. (2015) that students use different problem-solving styles in solving math problems.
SUMMARY, CONCLUSION, AND RECOMMENDATIONS
This chapter presents the summary of the major findings of the study, the conclusion, and the proposed recommendations for possible implementations.
Summary of Findings
The major findings of the study are the following:
- For the level of performance of students in solving math problem, the level of performance of students in terms of answering the 40-item Mathematics test has a mean of 60.84 with a descriptive equivalent of high. This indicates that the performance of students in mathematics is very good. Furthermore, it has an SD of 10.48. This demonstrates whether one’s scores on mathematical problem-solving style were extremely high or extremely low.
- For the level of mathematical problem-solving style, “feeling” got the highest mean of 3.48 with an SD of 0.98. This is followed by “sensing” with a mean of 3.42 and an SD of 0.97. Both indicators got a similar descriptive equivalent of high. On other hand, “intuitive” got the lowest mean of 3.26 and an SD of 1.01 with a descriptive equivalent of moderate. Furthermore, it has an overall mean of 3.38 and an SD of 0.99 with a descriptive equivalent of moderate.
- The statistical analysis shows that there is a strong positive correlation (r=0.733) between the mathematical problem-solving style and students’ performance. The p-value of the two variables is less than 0.05, indicating that the correlation is statistically significant. This means that there is a significant relationship between the students’ mathematical problem-solving style and their academic performance. As a result, the null hypothesis is rejected.
- The statistical analysis shows that there is no significant difference (t=211, p=0.9860) in the mathematical problem-solving style of students when grouped according to sex (male and female). The result indicates that the difference in mathematical problem-solving style between males and females is not statistically significant at the 0.05 level. Therefore, the researchers fail to reject the null hypothesis that there is no significant difference in the mathematical problem-solving style of students when grouped according to sex (male and female). Meanwhile, there is a significant difference (f=12.46, p=0.000) in the mathematical problem-solving style of students when grouped in accordance to different programs since the f-value is 12.46 and the p-value is 0.000 which is lesser than 0.05 alpha level of significance. This indicates the mathematical problem-solving style of students from BTVTED, BSAB, BSOA, and BPA is significantly different. Therefore, the null hypothesis is rejected.
- Based on the results of the study, here are the instructional intervention plan that can be proposed:
- For thinking and intuitive problem solvers – Provide explicit instruction on how to justify mathematical solutions using evidence and logical reasoning. Encourage intuitive problem-solvers to verbalize their thought process and explain how they arrived at their solution. Moreover, provide opportunities for students to develop their critical thinking skills through activities such as puzzles, brain teasers, and logic games. Encourage students to explain their reasoning and thought processes when solving problems.
- For sensing and feeling problem solvers – Provide opportunities for collaborative problem-solvers to work in pairs or small groups to solve problems. Encourage the students to take turns explaining their thought process and to ask questions to deepen their understanding. Provide opportunities for independent problem-solving and self-directed learning while allowing for collaborative work. Furthermore, provide support and guidance as needed to help students build their problem-solving skills. Gradually remove support as students become more confident and independent.
Conclusion
The findings from the study led the researcher to draw the following conclusions:
- Performance of students in solving math problems is very good.
- Mathematical problem-solving style of students is moderately observed.
- There is a significant relationship between mathematical problem-solving style and the performance of students.
- There is no significant difference in the mathematical problem-solving style of students when grouped according to sex. However, students’ mathematical problem-solving style is significantly different when grouped according to different programs.
- Understanding students’ different mathematical problem-solving styles can help instructors tailor their instruction to meet the needs of different learners and create a more inclusive classroom environment.
Recommendations
Based on the findings, analysis, and conclusion drawn in this study, the following recommendations were summarized:
- Students are encouraged to learn effectively and independently in solving mathematical tasks. They may discover that strengthening their different mathematical problem-solving style would boost their performance in math. This can be achieved by helping them discover their different problem-solving styles and identifying strategies that can assist them in solving mathematical problems. By doing so, they can improve their performance in mathematics and unleash their full potential in this subject area.
- Instructors, college administrators, and local college officials are urged to develop enrichment activities to help their students in developing their mathematical problem-solving styles. This is especially true when it comes to encouraging students to take the initiative, establish their own mathematical problem-solving strategy, set learning goals, and assess their abilities to specify the sources they need to learn, particularly in mathematics. Teachers may create engaging instructional intervention programs to deepen students’ interests in problem-solving in Mathematics. Furthermore, they can engage in and be creative with technology, mentoring, and coaching students who are having difficulty completing mathematical problems.
- The current study’s findings emphasize the relevance of problem-solving style in mathematics and provide ways to apply and improve it. The establishment of a curriculum and instructional strategy for applying it to students is required so that teachers and students can continue and maximize their mathematical problem-solving style. To ensure that these tactics are embedded in students, ongoing efforts will be required. To maximize students’ problem-solving style in Mathematics, STCAST instructors and administrators should work collaboratively to achieve the objective. They should ensure that the necessary materials, resources, activities, and differentiated instruction are available and used to meet students’ needs to be motivated in learning.
- Future research for developing other intervention programs needed to identify the factors that might improve the mathematical problem-solving style to enhance students’ performance.
REFERENCES
- Ahmed, W., van der Werf, G., Kuyper, H., & Minnaert, A. (2013). Emotions, Self-Regulated Learning, and Achievement in Mathematics: A Growth Curve Analysis. Journal of Educational Psychology, 105, 150-161.https://doi.org/10.1037/a0030160
- Ali, Norhidayah, Jusoff, Kamaruzaman, Ali, Syukriah, Mokhtar, Najah and Salamt, Azni Syafena Andin. (20 December 2009). ‘The Factors Influencing Students’ Performance at Universiti Teknologi MARA Kedah, Malaysia’. Canadian Research & Development Center of Sciences and Cultures: Vol.3 No.4.
- Altun, İ. (2003). The perceived problem-solving ability and values of student nurses and midwives. Nurse education today, 23(8), 575-584.
- Almagro, R.E., Montepio, H.C. & Tuquib, M.O. (2023). E-Learning Educational Atmosphere and Technology Integration as Predictors of Students’ Engagement: The Case of Agribusiness Program. Industry and Academic Research Review, 4 (1), 339-343.
- Almagro, R. E., EDIG, M. M. (2023). Motivated Strategies for Learning Mathematics as Influenced by Computer Attitude and Social Media Engagement of Students. Journal of Social, Humanity, and Education.
- Amran, M. S., Bakar, A. Y. A. (2020). We Feel, Therefore We Memorize: Understanding Emotions in Learning Mathematics Using Neuroscience Research Perspectives. Universal Journal of Educational Research, 8(11B),5943-5950. DOI: 10.13189/ujer.2020.082229.
- Badger, M. S., Sangwin, C. J., Hawkes, T. O., Burn, R. P., Mason, J., & Pope, S. (2012). Teaching Problem-Solving in Undergraduate Mathematics. Coventry, UK: Coventry University https://doi.org/10.1017/CBO9781107415324.004.
- Blackwell, L.S., Trzesniewski, K.H., & Dweck, C.S.(2007). Implicit theories of intelligence predict achievement across an adolescent transition: A longitudinal study and an intervention. Child Development, 78 (1), 246– 263. https://doi.10.1111/j.1467-8624.2007.00995.x
- Bradley, M. (2012). Problem-solving differences between men and women. Retrieved from http://www.healthguidance.org/entry/13967/1/ProblemSolving–Differences-Between-Men-and-Women.html
- Bishop, J. P. (2012). “She’s always been the smart one. I’ve always been the dumb one”: Identities in the mathematics classroom. Journal for Research in Mathematics Education, 43(1), 34. https://doi:10.5951/jresematheduc.43.1.0034
- Burkey, L. A., & Miller, M. K. (2005). Examining gender differences in intuitive decision making in the workplace. Gender and Behavior, 3, 252-268.
- Cribbs, J. D., Hazari, Z., Sonnert, G., & Sadler, P. M.(2015). Establishing an Explanatory Model for Mathematics Identity. Child Development, 86 (4),1048– 1062. https://doi.org/10.1111/cdev.12363
- Conner, M. G. (2000). Understanding the difference between men and women. Family Relations, 32, 567-573.IEA. (2016). The TIMSS 2015 International Results in Mathematics. In TIMSS & PIRLS International Study Center. Retrieved from http://timss2015.org/.
- Fernandez, M.L, Hadaway, N., & Wilson, J.W. (2017). Mathematical Problem Solving. http://jwilson.coe.uga.edu/emt725/PSsyn/PSsyn.html
- Foshay, R., & Kirkley, J. (2003). Principles for teaching problem solving. Plato Learning, 1–16. https://doi.org/10.1.1.117.8503&rep=rep1&type=pdf
- GANZON, W. J., & EDIG, M. M. . (2022). Time Management And Self-Directed Learning As Predictors Of Academic Performance Of Students In Mathematics. Journal of Social, Humanity, and Education, 3(1), 57–75. https://doi.org/10.35912/jshe.v3i1.1212
- Glass, G. V & Hopkins, K.D. (1984). Statistical Methods in Education and Psychology, 2nd Edition. Englewood Cliffs, NJ: Prentice-Hall.
- Ghodrati, M., Bavandian, L., Moghaddam, M. M., & Attaran, A. (2014). On the relationship between problem-solving trait and the performance on Ctest. Theory and Practice in Language Studies, 4(5), 1093-1100.
- Gürsan, S., & Yazgan, Y. (2020). Non-Routine problem-solving skills of ninth grade students: An experimental study. Academy Journal of Educational Sciences, 4(1), 23-29.
- Hafriani. (2018). Karakteristik Intuisi Mahasiswa UIN dalam Memecahkan Masalah Matematika Ditinjau Dari Kemampuan Berpikirnya. Pedagogik, Volume 1, Nomor 2
- Heris and Sumarmo. (2014). Penilaian Pembelajaran Matematika. P T Refika Aditama
- Hendriana, H., Rohaeti, E. E., &Sumarmo, U. (2017). Hard skills and soft skills mathematical. Bandung: Refika Aditama.
- Hsieh, Y.-P., & Lin, C.-H. (2006). Mathematical problem-solving style as a function of sensory preference. Educational Studies in Mathematics, 63(3), 321-335.
- Jung, J. Y., Youn, H. O., & Kim, H. J. (2007). Positive thinking and life satisfaction amongst Koreans. Yonsei Medical Journal, 48(3), 371-378.
- Khan, M. J., Younas, T., & Ashraf, S. (2016). Problem Solving Styles as Predictor of Life Satisfaction Among University Students. Pakistan Journal of Psychological Research, 31(1).
- Kneeland, S. (2001). Problem Çözme (N. Kalaycı, Çev.). Ankara: Gazi Kitabevi. Konan, N.(2013). Relationship between locus of control and problem-solving skills of high school administrators. International Journal of Social Sciences and Education, 3(3), 786-794.
- Macaso, K. M. J., & Dagohoy, R. G. (2022). Predictors of Performance in Mathematics of Science, Technology And Engineering Students of a Public Secondary School in The Philippines. Journal of Social, Humanity, and Education, 2(4), 311-326.
- Moon, J. (2008). Critical thinking: An exploration of theory and practice. New York: Routledge.
- Mustafić, M., Niepel, C., & Greiff, S. (2017). Assimilation and contrast effects in the formation of problem-solving self-concept. Learning and Individual Differences, 54, 82-91
- NCTM. (2000). Principles and Standards for School Mathematics. United States of America: NCTM.
- OECD. (2019). PISA 2018 Results: What Student Know and Can Do. https://doi.org/10.1787/5f07c754-en.
- Plaks, J. E., & Stecher, K. (2007). Unexpected improvement, decline, and stasis: A prediction confidence perspective on achievement success and failure. Journal of Personality and Social Psychology,93 (4), 667– 684. https://doi.org/10.1037/0022-3514.93.4.667
- Polya, G. (1957). How To Solve It: A New Aspect of Mathematical Method (Second). https://doi.org/10.2307/j.ctvc773pk.
- Rusdi, M., Fitaloka, O., Basuki, F. R., & Anwar, K. (2020). Mathematical Communication Skills Based on Cognitive Styles and Gender. International Journal of Evaluation and Research in Education, 9(4), 847-856.
- Saeed, R. (2015). Use of smartphones and social media in medical education: trends, advantages, challenges and barriers. Acta informatica medica, 27(2), 133.
- Surya, E., & Putri, F. A. (2017). Improving Mathematical Problem-Solving Ability and Self-Confidence of High School Students through Contextual Learning Model. Journal on Mathematics Education, 8(1), 85-94. http://dx.doi.org/10.22342/jme.8.1.3324.85-94
- Sutherland, L. (2002). Developing problem-solving expertise: the impact of instruction in a question analysis strategy. Learning and Instruction, 12(2), 155-187.
- Tan, L. (2014). Correlational study: W. F. Thompson Music in the social and behavioral sciences. Thousand Oaks: SAGE Publications, 1(3), 269-271.
- Taylor, G. R., & Mackenny, L. (2008). Improving human learning in the classroom: Theories and teaching practices. New York: Rowman & Littlefield Education
- Vicente, R. S., Flores, L. C., Almagro, R. E., Amora, M. R. V., & Lopez, J. P. (2023). The Best Practices of Financial Management in Education: A Systematic Literature Review. International Journal of Research and Innovation in Social Science, 7(8), 387-400.DOI: https://dx.doi.org/10.47772/IJRISS.2023.7827
- Wahono, B., Chang, C. Y., & Retnowati, A. (2020). Exploring a direct relationship between students’ problem-solving abilities and academic achievement: A STEM education at a coffee plantation area. Journal of Turkish Science Education, 17(2), 211-224.
- Wijayanti, A., Herman, T., & Usdiyana, D. (2017). The implementation of CORE model to improve students’ mathematical problem-solving ability in secondary school. Advances in Social Science, Education and Humanities Research, 57, 89–93. https://doi.org/10.2991/icmsed-16.2017.20.